BCSSS

International Encyclopedia of Systems and Cybernetics

2nd Edition, as published by Charles François 2004 Presented by the Bertalanffy Center for the Study of Systems Science Vienna for public access.

About

The International Encyclopedia of Systems and Cybernetics was first edited and published by the system scientist Charles François in 1997. The online version that is provided here was based on the 2nd edition in 2004. It was uploaded and gifted to the center by ASC president Michael Lissack in 2019; the BCSSS purchased the rights for the re-publication of this volume in 200?. In 2018, the original editor expressed his wish to pass on the stewardship over the maintenance and further development of the encyclopedia to the Bertalanffy Center. In the future, the BCSSS seeks to further develop the encyclopedia by open collaboration within the systems sciences. Until the center has found and been able to implement an adequate technical solution for this, the static website is made accessible for the benefit of public scholarship and education.

A B C D E F G H I J K L M N O P Q R S T U V W Y Z

BAKER'S TRANSFORMATION 2)

← Back

A topological transformation which helps to visualize the chaotic fractalization of processes trajectories in complex systems.

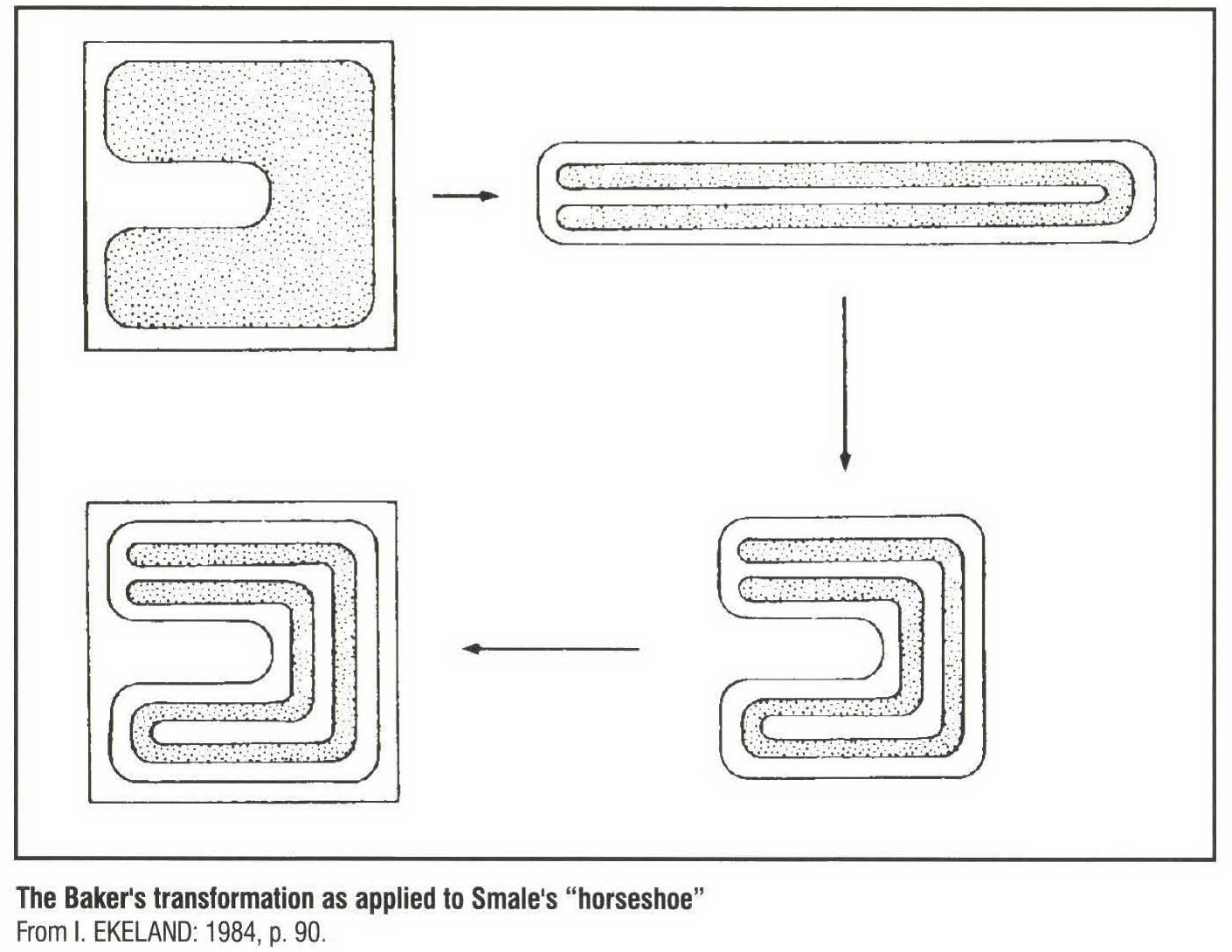
I. PRIGOGINE and Y. ELSKENS describe the Baker transformation of a unit square as "a piecewise linear area-preserving mapping" (1986, p.211)

PRIGOGINE describes it as follows: "(it) owes its name to the association with the kneading of dough… Beginning with a square, we first flatten it into a rectangle. Then we fold one half of the rectangle on top of the other half to form a square again. This set of operations… may be repeated as many times as one likes. Each time the surface of the square is broken up and redistributed. The square here corresponds to the phase space. The baker transformation takes each point from its original positions to a new, but perfectly well defined position… Although the series of points obtained in this way is "deterministic", the system displays in addition some irreducibly statistical aspects" (1980, p.77).

As explained by J. CASTI: "The stretching and folding operations are complementary, in the sense that the first separates points, while the second tries to bring them back together again – but with new neighbors" (1994, p.91).

He adds: "There are two directions in which the points can move: up/down and left/right. So there are two rates of separation, one in the vertical direction, the other in the horizontal" (p. 100).

The baker's transformation is a model of how a deterministic process can produce a chaotic behavior.

Categories

  • 1) General information
  • 2) Methodology or model
  • 3) Epistemology, ontology and semantics
  • 4) Human sciences
  • 5) Discipline oriented

Publisher

Bertalanffy Center for the Study of Systems Science(2020).

To cite this page, please use the following information:

Bertalanffy Center for the Study of Systems Science (2020). Title of the entry. In Charles François (Ed.), International Encyclopedia of Systems and Cybernetics (2). Retrieved from www.systemspedia.org/[full/url]

We thank the following partners for making the open access of this volume possible:

© 2020 BCSSS Contact/Imprint